Quite a few times, you would have tried to measure something against a scale, and there is a possibility that each time the value might have been somewhat different from what it was previously. And, that is particularly how the uncertainty in a measurement operation comes into being.
When measuring the length, size, volume, or some other physical property of an object, the errors that might come along with that significant measures are needed to be taken into account. And, that is particularly what brings us to the question if the uncertainties can be sorted out by using simple mathematical operations or not.
Step to Step Guide to Calculate the Uncertainty in a Measurement
Precision and accuracy in mathematical operations are really necessary, and for that precision, it becomes important to take into account the errors or faults that might come along with that measurement. And, following are the steps that you can take to reach an effective conclusion easily:
1. Find the Reason for the Uncertainty
There are multiple reasons why complications can arise when you are trying to take an accurate measurement of something. And the major one in these is the errors in the measuring devices or the scales. This problem stands true even for the centimeter scales. And, for the other scientific devices, this is termed the zero error or the error that arises when the zero of one scale does not align with that of the other scale.
2. Calculate the Terms of the Error
The errors in measurement during an operation are needed to be in terms of the least count of the particular scale that is being used for the measurement. The uncertainty that is significantly out of reach compared to these bounds (or far surpassing the measure of least count) is not regarded as correct.
The least count is the smallest unit that can be measured with a scale or means of measurement with the greatest precision.
least count = difference between the two consecutive scalar valuesnumber of small-scale divisions between the two valuesleast count = difference between the two consecutive scalar valuesnumber of small-scale divisions between the two values
For the centimeter scales that we are accustomed to using, the value of the least count is 0.1cm.
3. Extent of Measurement
Scientifically as well as mathematically, an uncertainty of up to 20% and 10%, respectively, of the actual value is regarded as correct in these theories. An error that exceeds that measure is regarded as completely incorrect, and the conclusion to these kinds of terms is not supported on any grounds.
For example,
Consider a ruler of 15cm in length. And, you are asked to trace a straight line along that scale of 30cm. then, you will need to use that scale twice or maybe even more than two times. And, in these turns, you are bound to make an error in that measurement. But, it will still be regarded as correct as long as it is within 3cm of the actual value measured.
4. Types of Uncertainties
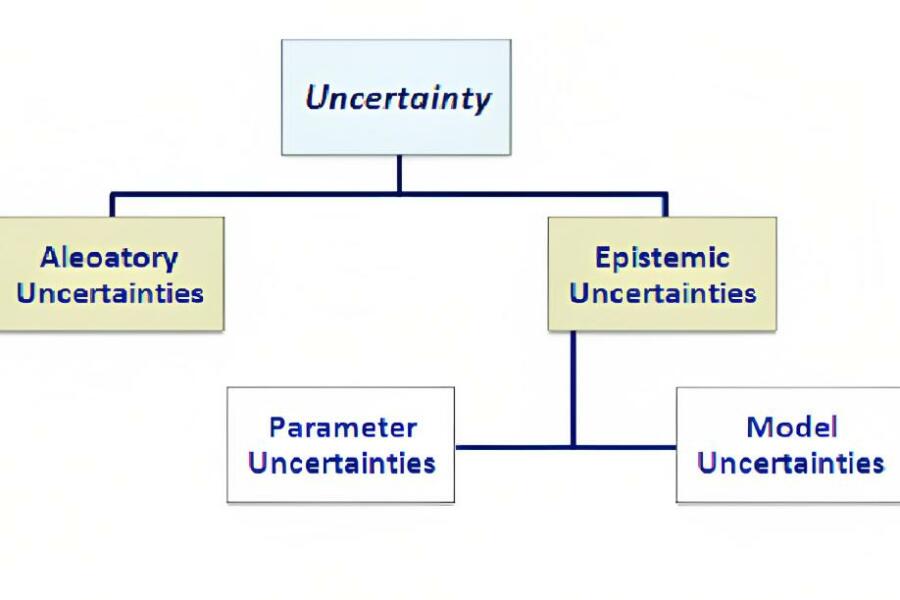
During the procedure of measurement, the uncertainties can be positive as well as negative. It is the same as the zero counts of an instrument. It signifies that when measuring a value, you can measure the value to be more than the actual one, or maybe even less than the actual value. Hence, it works both ways and is needed to be corrected in both ways.
5. Correction of Uncertainty
If there is a positive uncertainty in the measurement, it will mean that you have a value that is more than the actual value. To make it right you will need to subtract the measure of the uncertainty from the calculated value.
If the uncertainty is negative, then you will need to add the difference between the actual value and the recorded value to the recorded value. This will help you in arriving at an accurate conclusion.
6. Representation of Uncertainty
The uncertainty for measurement is denoted by (±). Suppose that a problem is written as (x ± y), then it means that the solution to that question can vary from (x + y) to (x – y), depending on the various variables or sources of errors that might be included in the process of calculation.
The symbol of uncertainty signifies that the value of the result can vary in the positive and negative direction to a certain extent.
Correction of Uncertainties
1. Take Multiple Measurements
The most effective way to counter the problem of uncertainty will be to measure a value over and over again till you achieve the value that is the closest to the actual reading. This is known to be significantly effective, as it is known to reduce the margin of uncertainty with each successive try.
2. Taking The Mean of Data
If you are not provided with the actual value, then the best course of action will be to account for all the values that you measure. But, this will only leave you with many outputs for a single operation. Therefore, the average of the obtained data is needed to be taken for more effectiveness.
3. Net Uncertainty
After you have calculated the mean for the obtained data, then subtract the mean from all the values that you measured for the operation. This will leave you with certain uncertainties for each observation that you calculated on your own. Now, take the mean of all these obtained uncertainties, and that will help you in getting to the mean uncertainty of that data.
Operations on Uncertainties
Mathematical or arithmetic operations work on the uncertainties the same way that they do when applied to numbers. If two values, both with uncertainties, are multiplied or added, then the actual value will be operated with the actual value, and the uncertainty will be operated with the other uncertainty.
For example,
(x y) + (a
b) = (x + a)
(y + b)
(x y) * (a
b) = (x * a)
(y * b)
You need to keep in mind that, if the uncertainty is in decimals, then upon operation, the uncertainty will still be in the same number of decimals as before. The decimal point will not be displaced.
Also read: Guide to Convert Degree to Radian























































